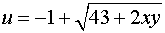Example 6.6.2: Solve 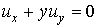 ,
,
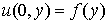 using method of characteristics.
using method of characteristics.
Solution: The characteristic equation is
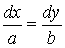 (but a=1, b=y)
(but a=1, b=y)
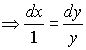
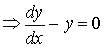
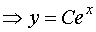
where C is a constant.
If the solution passes through (x0,
y0) (that is x0is
a point on the initial curve) then 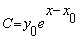 which gives
which gives
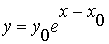
The solution along this characteristic is since
the initial conditions are given at x=0 and each
characteristic has to pass through the initial solution to have
the given PDE a a unique solution we have 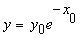 .
Hence the solution to the given PDE can be written as
.
Hence the solution to the given PDE can be written as
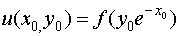 or
or

General Theory of Characteristic Curves
The general theory of characteristic curves should encompass more general equations
and not treat the variables x and y in an unsymmetrical
way. Furthermore, the solution may not be constant along a characteristic curve;
it may only satisfy ordinary differential equation along a characteristic.
Consider the equation

.................
(6.6.9)
in which a, b and c are functions
of x, y and u. Suppose that the value
of the solution is known at a point (x0, y0).
This could be the result of prior calculations or a boundary value that had
been prescribed. We shall indiacate now how additional solution values of (6.6.9)
can be obtained by integrating some ordinary differntial equations. Consider
this system of three ordinary differential equations with initial conditions:
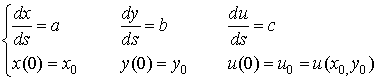
................. (6.6.10)
To produce a table of x(s), y(s), u(s)
starting at s=0. One can integrate this system numerically. It
is not hard to verify that this table will provide solution points of the partial
diferential equation (6.6.9). Indeed.
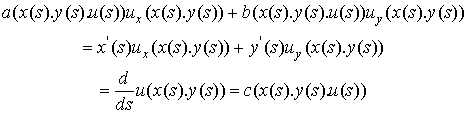
(In this calculation primes indicate differentiation with respect
to s)
If the values of u(x,y) have been prescribed along
some curve  (not
a characteristic curve) then in principle one can start at any point of
(not
a characteristic curve) then in principle one can start at any point of  ,
integrate system (6.6.10), and obtain additional values of u(x, y)
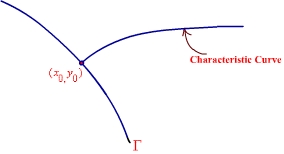
along arcs. (See figure 6.6.1 for help in understanding this idea). The arcs
obtained in this process are characteristic curves for Equation (6.6.9).
,
integrate system (6.6.10), and obtain additional values of u(x, y)
along arcs. (See figure 6.6.1 for help in understanding this idea). The arcs
obtained in this process are characteristic curves for Equation (6.6.9).
Example 6.6.3: Consider the
partial differential equation
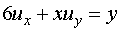
With u(3, 3) =4, what value of u(15,
21) is obtained by following a characteritic curve?
Solution
The characteristic curve through the initial point is governed
by the initial-value problem
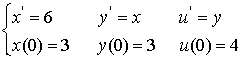
On integrating these equations, we obtain
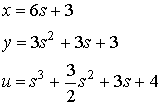
Letting s = 2, we arrive at x = 15,
y = 21, u = 24.
Example 6.6.4: Use the method
of characteristics to find a solution of the boundary-value problem
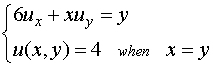
Solution : Let us find the characteristic curves passing through
the point (x, y)=(r, r), r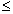 6.
6.
We solve the system
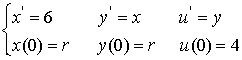 '
'
and obtain
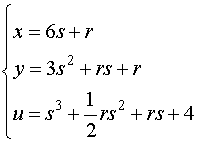
If (x, y) is a given point in the plane, we try to determine
a corresponding (r, s) using the first two equations in (23). The result
of this calculation is
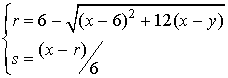
With the values of r and s at hand,
the value of u at(x, y) can be computed from the
third equation in (23). Thus for example, if (x,y)=(15,21), then
(r,s)=(3,2) and u=24 as in Example 6.6.4.
Example 6.6.5: Solve the boundary
value problem
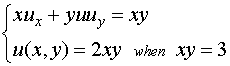
by the method of characteristics.
Solution: The equations for
the characteristics are
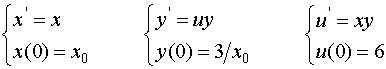
Since
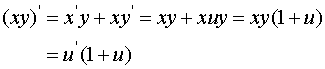
Integrating with respect to s given

but u(x, y) = 2xy when xy=3 given
C=-21